Two particles, $A$ and $B$, are attached to the ends of a light inextensible string, which passes over a smooth pulley. Particle $A$ has mass $8 \mathrm{kg}$ and $B$ has mass $5 \mathrm{kg}$. Both particles are held $1.2 \mathrm{m}$ above the ground. The system is released from rest and the particles move vertically.

When particle $A$ hits the ground, it does not bounce. Find the maximum height reached by particle $B$.

Two particles, $A$ and $B$, are attached to the ends of a light inextensible string, which passes over a smooth pulley. Particle $A$ has mass $8 \mathrm{kg}$ and $B$ has mass $5 \mathrm{kg}$. Both particles are held $1.2 \mathrm{m}$ above the ground. The system is released from rest and the particles move vertically.

When particle $A$ hits the ground, the string is cut. Find the total time from being released from rest until $B$ hits the ground.

A particle, $P$, moves on a straight track. It has displacement $s \mathrm{m}$ from a point, $O$, at time $t \mathrm{s}$, given by $s=0.12t+10-0.01t^3$ for $t>0$.

Find the time when the particle is first stationary.

A particle, $P$, moves on a straight track. It has displacement $s \mathrm{m}$ from a point, $O$, at time $t \mathrm{s}$, given by $s=0.12t+10-0.01t^3$ for $t>0$. Find the total displacement in first $10 sec$.

A particle, $P$, moves on a straight track. It has displacement $s \mathrm{m}$ from a point, $O$, at time $t \mathrm{s}$, given by $s=0.12t+10-0.01t^3$ for $t>0$. Particle $Q$ moves on a track parallel to particle $P$. The acceleration, $a \mathrm{m}{ \mathrm{s}}^{-2}$, of $Q$ is given by $a=0.4-0.06t$. Both particles come to rest alongside each other. Find the displacement of $Q$ from $O$ after $10 \mathrm{s}$.

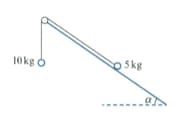
Two particles of masses $5 \mathrm{kg}$ and $10 \mathrm{kg}$ are connected by a light inextensible string that passes over a fixed smooth pulley. The $5 \mathrm{kg}$ particle is on a rough fixed slope which is at an angle of $\alpha$ to the horizontal, where $\tan \alpha =\frac{3}{4}$. The $10 \mathrm{kg}$ particle hangs below the pulley (see diagram). The coefficient of friction between the slope and the $5 \mathrm{kg}$ particle is $\frac{1}{2}$. The particles are released from rest. Find the acceleration of the particles and the tension in the string.

A particle of mass $0.1 \mathrm{kg}$ is released from rest on a rough plane inclined at $20°$ to the horizontal. It is given that, $5$ seconds after release, the particle has a speed of $2 \mathrm{m}{ \mathrm{s}}^{-1}$.

Find the acceleration of the particle and hence show that the magnitude of the frictional force acting on the particle is $0.302 \mathrm{N}$, correct to $3$ significant figures.

A particle of mass $0.1 \mathrm{kg}$ is released from rest on a rough plane inclined at $20°$ to the horizontal. It is given that, $5$ seconds after release, the particle has a speed of $2 \mathrm{m}{ \mathrm{s}}^{-1}$.

Find the coefficient of friction between the particle and the plane.

A particle $P$ moves in a straight line. It starts at a point $O$ on the line and at time $t \mathrm{s}$ after leaving $O$ it has a velocity $v \mathrm{m}{ \mathrm{s}}^{-1}$, where$v=6t^2-30t+24$.

Find the set of values of $t$ for which the acceleration of the particle is negative.