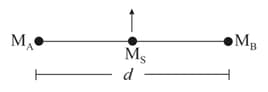
Two planets $A$ and $B$ are fixed at a distance $d$ from each other as shown in the figure. If the mass of $A$ is $M_{\mathit{A}}$ and that of $B$ is $M_{\mathit{B}}$, then find out the minimum velocity of a satellite of mass $M_{\mathit{s}}$ projected from the mid-point of two planets to infinity. 

Two identical stars of mass $M$, orbit around their centre of mass. Each orbit is circular and has radius $R$, so that the two stars are always on opposite sides on a diameter. 

(a) Find the gravitational force of one star on the other. 

(b) Find the orbital speed of each star and the period of the orbit.

(c) Find their common angular speed. 

(d) Find the minimum energy that would be required to separate the two stars to infinity.

(e) If a meteorite passes through this centre of mass perpendicular to the orbital plane of the stars. What value must its speed exceed at that point if it escapes to infinity from the star system?

Two earth satellites $A$ and $B$ each of equal mass are to be launched into circular orbits about the earth’s centre. Satellite $A$ is to orbit at an altitude of$6400 \mathrm{km}$ and $B$ at $19200 \mathrm{km}$. The radius of the earth is $6400 \mathrm{km}$. Determine-

(a) the ratio of the potential energy.

(b) the ratio of kinetic energy.

(c) Which one has the greater total energy.

Saturn is about six times farther from the Sun than Mars. Which planet has:

(a) the greater period of revolution? 

(b) the greater orbital speed and

(c) the greater angular speed?

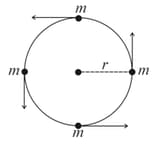
Four similar particles of mass $m$ are orbiting in a circle of radius $r$ in the same direction and same speed because of their mutual gravitational attractive force as shown in the figure. Speed of a particle is given by, 

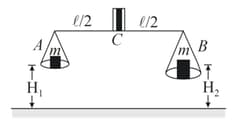
Two blocks of masses $\mathrm{m}$ each are hung from a balance as shown in the figure. The scale pan $\mathrm{A}$ is at height ${\mathrm{H}}_1$ whereas scale pan $\mathrm{B}$ is at height ${\mathrm{H}}_2$. Net torque of weights acting on the system about point $\text{'}\mathrm{C}\text{'}$, will be (length of the rod is $\mathrm{l}$ and${\mathrm{H}}_1 \& {\mathrm{H}}_{2 }\mathrm{are} << \mathrm{R}) ({\mathrm{H}}_1 > {\mathrm{H}}_2)$