Two wires of same material are hanging as shown in the figure. Breaking stress is equal for both the wires.

Assertion: If mass '${\mathrm{m}}_2$' increases, then upper wire will break first.

Reason: Breaking load of two wires of same material can be different.

A uniform bar of square cross-section is lying along a frictionless horizontal surface. A horizontal force is applied to pull it from one of its ends. Then,

A light rod of length $200 \mathrm{cm}$ is suspended from the ceiling horizontally by means of two vertical wires of equal length tied to its end. One of the wires is made of steel and has area of cross-section $0.1$ ${\mathrm{cm}}^2$ and the other of brass of cross-sectional area $0.2 {\mathrm{cm}}^2.$ An object of mass $\mathrm{m}$ is hung to the rod. $\left({\mathrm{Y}}_{\text{steel }}=2\times {\mathrm{Y}}_{\text{brass }}\right)$

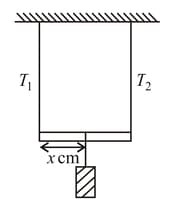
At which distance from one end of the rod should a weight be hung to produce equal strains in both the wires?

A steel wire of diameter $d=1\mathrm{mm}$ is stretched horizontally between $2$ clamps located at distance $l=2 \mathrm{m}$ from each other. A weight of mass $\mathrm{m}=0.25 \mathrm{kg}$ is suspended from midpoint $O$ of the wire. What will the resulting descent of the point $\mathrm{O}$ be in $\mathrm{cm}$? (Here, the modulus of elasticity of steel is $E_{\mathrm{steel}}=2\times {10}^{11} \mathrm{N} {\mathrm{m}}^2$)

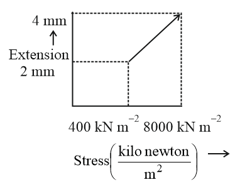
In the determination of Young's modulus of elasticity of a wire, a force is applied and extension is recorded. Initial length of wire is $1 \mathrm{m}$. The curve between extension and stress is depicted, then Young's modulus of wire will be:

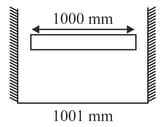
A rod of length $1000 \mathrm{mm}$ and coefficient of linear expansion $\alpha ={10}^{-4}$ per degree is placed symmetrically between fixed walls separated by $1001 \mathrm{mm}$. Young's modulus of the rod is ${10}^{11}\mathrm{N} {\mathrm{m}}^{-2}$. If the temperature is increased by $20°\mathrm{C},$ then the stress developed in the rod is (in $\mathrm{N} {\mathrm{m}}^2$)

Two forces ${\mathrm{F}}_1$ and ${\mathrm{F}}_2$ act on a thin uniform elastic rod placed in space. Force ${\mathrm{F}}_1$ acts at right end of the rod and ${\mathrm{F}}_2$ acts exactly at centre of rod as shown (both forces act parallel to length of the rod).

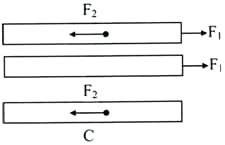
(i) ${\mathrm{F}}_1$ causes extension of rod while ${\mathrm{F}}_2$ causes compression.
(ii) ${\mathrm{F}}_1$ and ${\mathrm{F}}_2$ both cause extension of rod.
(iii) ${\mathrm{F}}_1$ causes extension of rod while ${\mathrm{F}}_2$ does not change length of rod. The correct order of True (T) or False (F) statements is

A rod $1\mathrm{m}$ long is $10 {\mathrm{cm}}^2$ in area for a portion of its length and $5 {\mathrm{cm}}^2$ in area for the remainder. The strain energy of this stepped bar is $40\%$ of that of a bar $10 {\mathrm{cm}}^2$ in area and $1$ $\mathrm{m}$ long under the same maximum stress. What is the length of the portion $10 {\mathrm{cm}}^2$ in area?

The atmospheric pressure on the earth's surface is $\mathrm{P}$ in $\mathrm{M}.\mathrm{K}.\mathrm{S}$. units. A table of area $2 {\mathrm{m}}^2$ is tilted at $45°$ with the horizontal. The force on the table due to the atmosphere is ($\mathrm{stress} \times \mathrm{area}$).