What is angle of deviation due to reflection?

1. Laws of Reflection:

(i) The incident ray $\left(AB\right)$, the reflected ray $\left(BC\right)$ and normal $\left(NB\right)$ to the surface $\left(SS\text{'}\right)$ of reflection at the point of incidence $\left(B\right)$ lie in the same plane. This plane is called the plane of incidence (also plane of reflection).

(ii) The angle of incidence (the angle between normal and the incident ray) and the angle of reflection (the angle between the reflected ray and the normal) are equal ∠i=∠r.

2. Object:

(i) Real: Point from which rays actually diverge.

(ii) Virtual: Point towards which rays appear to converge.

3. Image:

Image is decided by reflected or refracted rays only.

The point image for a mirror is that point towards which the rays reflected from the mirror, actually converge (real image).

From which the reflected rays appear to diverge (virtual image).

4. Characteristics of reflection by a plane mirror:

(i) The size of the image is the same as that of the object.

(ii) For a real object the image is virtual and for a virtual object the image is real.

(iii) For a fixed incident light ray, if the mirror be rotated through an angle θ the reflected ray turns through an angle 2θ in the same sense.

(iv) Number of images $\left(n\right)$ in inclined mirror: Find 360θ=m,

(a) If m even, then n=m-1, for all positions of the object.

(b) If m odd, then $n=m,$ If object not on bisector and n=m-1, If object at bisector

(c) If m fraction then n=nearest even number

5. Spherical mirrors:

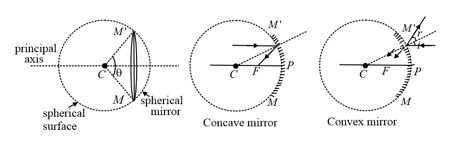
(i) Paraxial rays: Rays which form a very small angle with axis are called paraxial rays. All formulae are valid for paraxial rays only.

(ii) Sign convention:We follow cartesian coordinate system convention, according to which the pole of the mirror is the origin.

The direction of the incident rays is considered as positive x- axis. Vertically up is the positive y-axis.

All distances are measured from the pole.

(iii) Mirror formula: 1f=1v+1u

f=x-coordinate of focus

$u=x-$coordinate of object

v=x -coordinate of image

Note: Valid only for paraxial rays.

(iv) Transverse(or lateral) magnification: m=h2h1=-vu

$h_2=y-$coordinate of image $h_1=y-$coordinate of the object

(both perpendicular to the principal axis of mirror)

(v) Longitudinal magnification:

$m\text{'}=\frac{\mathrm{ }\mathrm{Length}\mathrm{ }\mathrm{of}\mathrm{ }\mathrm{image}}{\mathrm{ }}$

for small objects $m\text{'}=-m^2$, $m=$ transverse magnification.

(vi) Velocity of image of moving object (spherical mirror):

(a) Velocity component along axis (Longitudinal velocity)

When an object is coming from infinite towards the focus of concave mirror

$\because \frac{1}{v}+\frac{1}{u}=\frac{1}{f}\hspace{1em}\therefore -\frac{1}{v^2}\frac{\mathrm{d}v}{\mathrm{d}t}-\frac{1}{u^2}\frac{\mathrm{d}u}{\mathrm{d}t}=0 \Rightarrow {\overrightarrow{v}}_{\mathrm{IM}}=-\frac{v^2}{u^2}{\overrightarrow{v}}_{\mathrm{OM}}=-m^2{\overrightarrow{v}}_{\mathrm{OM}}$

$v_{\mathrm{IM}}=\frac{\mathrm{d}v}{\mathrm{d}t}=$ velocity of image with respect to mirror

$v_{\mathrm{OM}}=\frac{\mathrm{d}u}{\mathrm{d}t}=$ velocity of object with respect to mirror.

(vii) Newton's formula:

Applicable to a pair of real objects and real image position only. They are called conjugate positions or foci, $X_1 \text{and} X_2$ are the distance along the principal axis of the real object and real image respectively from the principal focus.

$X_1{X}_2=f^2$

(viii) Optical power: Optical power of a mirror (in Diopters), $P=-\frac{1}{f}$

where f=focal length (in meters) with sign.

6. Laws of Refraction:

(i) Incident ray, refracted ray and normal always lie in the same plane.

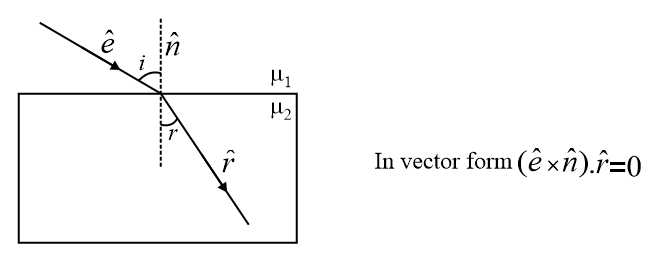
(ii) Snell's law: The product of refractive index and sine of angle of incidence at a point in a medium is constant. ${\mu }_1\sin i={\mu }_2\sin r$,

$\frac{\sin i}{\sin r}={ }_1{\mu }_2=\frac{{\mu }_2}{{\mu }_1}=\frac{v_1}{v_2}=\frac{{\lambda }_1}{{\lambda }_2}$ 

In vector form, ${\mu }_1\left|\hat{\mathrm{e}}\times \hat{\mathrm{n}}\right|={\mu }_2\left|\hat{\mathrm{r}}\times \hat{\mathrm{n}}\right|$

Note: Frequency of light does not change during refraction.

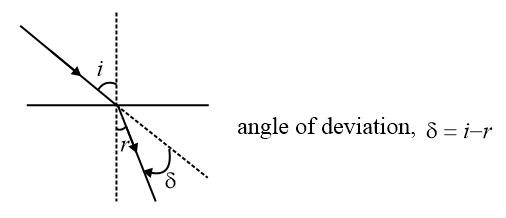
7. Deviation of a ray due to refraction:

8. Refraction through a parallel slab:

Emerged ray is parallel to the incident ray, if the medium is same on both sides.

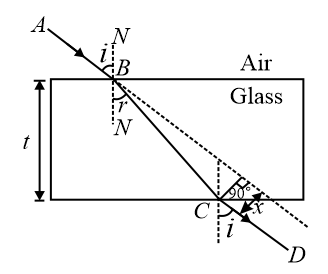
Lateral shift, $x=\frac{t\sin \left(i\right)\left(-\right)}{\left(r\right)}; t=$ thickness of slab

Note: Emerged ray will not be parallel to the incident ray if the medium on both the sides are different.

9. Apparent depth of submerged object:

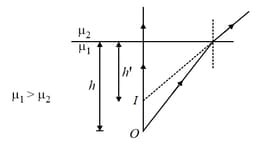
For near normal incidence, h'=μ2μ1h. Here, $\left(h\text{'}<h\right)$

$\mathrm{\Delta }x=$ Apparent shift =t1-1μ

Note: hand h' are always measured from the surface.

10. Critical angle & total internal reflection(TIR):

(i) Conditions of TIR:

(a) Ray is going from denser to rarer medium.

(b) Angle of incidence should be greater than the critical angle $\left(i>C\right)$.

Critical angle, $C={\sin }^{-1}\frac{{\mu }_{\mathrm{R}}}{{\mu }_{\mathrm{D}}}={\sin }^{-1}\frac{v_{\mathrm{D}}}{v_{\mathrm{R}}}={\sin }^{-1}\frac{{\lambda }_{\mathrm{D}}}{{\lambda }_{\mathrm{R}}}$

(ii) Some Illustrations of Total Internal Reflection:

(a) Sparkling of diamond: The sparkling of diamond is due to total internal reflection inside it. As the refractive index for a diamond is $2.5{\text{. So,}}^{ }C=24°\text{.}$ Now the cutting of diamond are such that $i>C$. So TIR will take place again and again inside it. The light which beams out from a few places in some specific directions makes it sparkle.

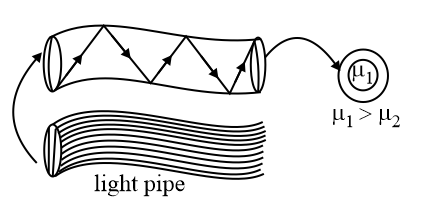
(b) Optical Fiber: In it light through multiple total internal reflections is propagated along the axis of a glass fiber of radius of few microns in which index of refraction of core is greater than that of surroundings (cladding).

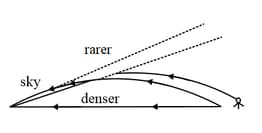
(c) Mirage and looming: Mirage is caused by total internal reflection in deserts where due to heating of the earth, refractive index of air near the surface of earth becomes lesser than above it. Light from distant objects reaches the surface of earth with $i>{\theta }_{\mathrm{c}}$ so that TIR will take place and we see the image of an object along with the object as shown in figure.

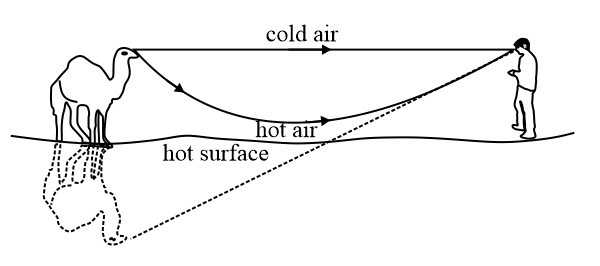
Similar to 'mirage' in deserts, in polar regions 'looming' takes place due to TIR. Here $\mu$ decreases with height and so the image of an object is formed in air if $\left(i>C\right)$ as shown in figure.

11. Refraction through a prism:

δ=i+e-A, r+r'=A Variation of δ versus i:

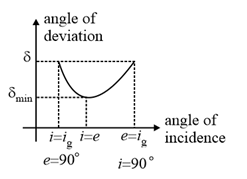
There is one and only one angle of incidence for which the angle of deviation is minimum.

When $\delta ={\delta }_m$${ }_{}$ then $\hspace{0.17em}$$i=e \& r=r^{\text{'}},$ the ray passes symmetrically about the prism, &

then $\mu =\frac{\sin \left[\frac{A}{}\right]\left[\frac{+\delta m}{2}\right]}{\sin \left[A/2\right]}\frac{\left[ \right]}{},$ where $\mu =$ absolute R.I. of glass.

Note: When the prism is dipped in a medium then, $\mu =R.I$. of glass w.r.t. medium.

Note: For a thin prism $\left(A\le 10°\right)\text{;} \delta =\left(\mu -1\right)A$

12. Dispersion of Light:

The angular splitting of a ray of white light into a number of components when it is refracted in a medium other than air is called Dispersion of Light.

Angle of Dispersion: Angle between the rays of the extreme colours in the refracted (dispersed) light is called Angle of Dispersion, θ=δv-δr

Dispersive power $\left(\omega \right)$ of the medium of the material of prism.

For small-angled prism, $\left(A\le 10°\right); \omega =\frac{{\delta }_{\mathrm{v}}-}{{\delta }_{\mathrm{r}}}=\frac{{\mu }_{\mathrm{v}}-}{{\mu }_{\mathrm{r}}}; \mu =\frac{{\mu }_{\mathrm{v}}+{\mu }_{\mathrm{r}}}{2}$,

${\mu }_{\mathrm{v}}, {\mu }_{\mathrm{r}} \& \mu$ are R.I. of material for violet, red & yellow colours respectively.

13. Combination of Two Prisms:

(i) Achromatic Combination: It is used for deviation without dispersion.

Condition for this, $\left({\mu }_{\mathrm{v}}-\right)\left({\mu }_{\mathrm{r}}\right)A=-\left(\mu {\text{'}}_{\mathrm{v}}-\right)\left(\mu {\text{'}}_{\mathrm{r}}\right)A\text{'}$

Net mean deviation, ${\delta }_{\mathrm{net}}=\left[\frac{{\mu }_{\mathrm{v}}+{\mu }_{\mathrm{r}}}{2}-1\right]A-\left[\frac{\mu {\text{'}}_{\mathrm{v}}+\mu {\text{'}}_{\mathrm{r}}}{2}-1\right]A\text{'}$ or $\omega \delta +\omega \text{'}\delta \text{'}=0$,

where $\omega \text{and}$$\omega \text{'}$are dispersive powers for the two prisms $\& \delta , \delta \text{'}$ are the mean deviation.

(ii) Direct Vision Combination: It is used for producing dispersion without deviation.

Condition for this $\left[\frac{{\mu }_{\mathrm{v}}+{\mu }_{\mathrm{r}}}{2}-1\right]A=-\left[\frac{\mu {\text{'}}_{\mathrm{v}}+\mu {\text{'}}_{\mathrm{r}}}{2}-1\right]A\text{'}$

Net angle of dispersion $=\left({\mu }_{\mathrm{v}}-\right)\left({\mu }_{\mathrm{r}}\right)A-\left(\mu {\text{'}}_{\mathrm{v}}-\right)\left(\mu {\text{'}}_{\mathrm{r}}\right)A\text{'}$

14. Refraction at the spherical surface:

μ2v-μ1u=μ2-μ1R

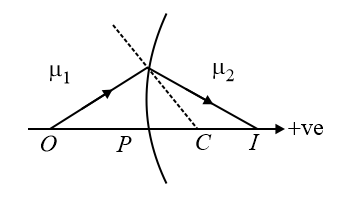
$v, u \& R$are to be kept with sign as

v=PI, u=-PO and R=PC

Note: (Radius is with sign)

Magnification, m=μ1vμ2u

15. Lens Formula:

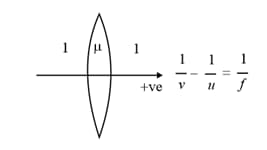
Lateral magnification: m=vu

Lens Maker’s formula: $\frac{1}{f}=\left(\mu -1\right)\left(\frac{1}{R_1}-\frac{1}{R_2}\right)$

16. Power of Lenses:

The Reciprocal of focal length in meters is known as the power of a lens.

Power of lens: $P=\frac{1}{f\left(m\right)}=\frac{100}{f\left(\mathrm{cm}\right)}$ dioptre

$\mathrm{SI}$ unit: dioptre $\left(D\right)$

17. Combination of Lenses:

(i) Two thin lens are placed in contact to each other,

(ii) Power of combination, $P=P_1+P_2 \Rightarrow \frac{1}{f}=\frac{1}{f_1}+\frac{1}{f_2}$

18. Newton's Formula:

Use sign convention when solve numerical using Newton's Formula.

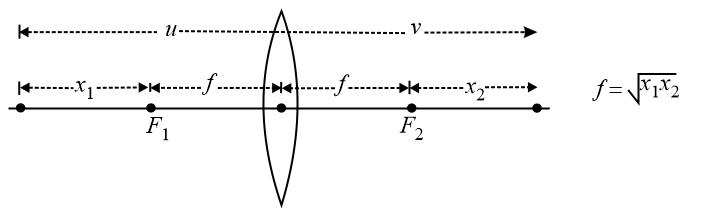
$x_1=$distance of the object from focus; $x_2=$distance of the image from focus.

19. Displacement Method:

It is used for the determination of the focal length of the convex lens in the laboratory. A thin convex lens of focal length f is placed between an object and a screen fixed at a distance $D$ apart.

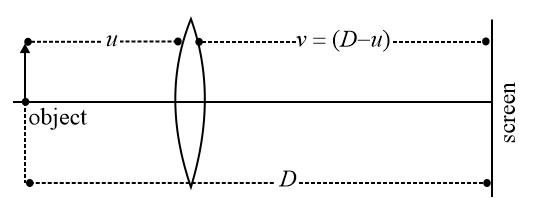
(i) For D<4f: u will be imaginary. Hence, physically no position of the lens is possible.

(ii) For D=4f: u=D2=2f, so only one position of the lens is possible and since v=D-u=4f-2f=u=2f

(iii) For $D>4f: u_1=\frac{D-\sqrt{D\left(D-4f\right)}}{2}$ and $u_2=\frac{D+\sqrt{D\left(D-4f\right)}}{2}$

So, there are two positions of lenses for which real images will be formed on the screen.

For two positions of the lens distances of object and image are interchangeable.

So, u1=D-x2=v2 and v1=D+x2=u2

m1=I1O=v1u1=D+xD-x and m2=I2O=v2u2=D-xD+x

Now, m1×m2=D+xD-x×D-xD+x ⇒I1I2O2=1⇒O=I1I2

20. Silvering of one surface of the lens :

$\left[\text{use} P_{\mathrm{eq}}=2P_{\mathrm{i}}+P_{\mathrm{m}}\right]$

When the plane surface is silvered,

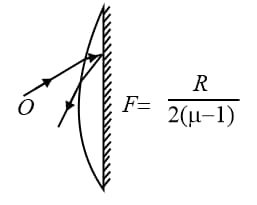
When the convex surface is silvered,

21. Optical instruments:

(i) For Simple microscope:

Magnifying power when the image is formed at $D$, $MP=1+D/f$

When the image is formed at infinity, MP=D/f

(ii) For Compound microscope: $MP=-\frac{v_0}{u_0}\left(\frac{D}{u_e}\right)$

Magnifying power when the final image is formed at $D$, $MP=-\frac{v_0}{u_0}\left(1+\frac{D}{f_e}\right)$

Tube length, $L=v_0+\left|u_e\right|$

When the final image is formed at infinity, MP=-v0u0×Df0 and L=v0+fe

(iii) Astronomical Telescope: MP=-f0ue

Magnifying power when final image is formed at $D$, MP=-f0fe1+feD

Tube length, $L=f_0+u_e^{ }$

When the final image is formed at infinity, MP=-f0fe and L=f0+f

(iv) For terrestrial telescope: MP=f0fe and L=f0+fe+4f

(v) For Galilean telescope: MP=f0fe & L=f0-fe

(vi) Lens camera: Time of exposure $\propto \frac{1}{{\left(\mathrm{aperture}\right)}^2}, f-\mathrm{number}=\frac{ \mathrm{focal} \mathrm{length} }{\mathrm{aperture}}$

(vii) For myopia or short-sightedness or nearsightedness: $\frac{1}{ F.P. }-\frac{1}{\mathrm{object}}=\frac{1}{f}=P$

(viii) For long-sightedness or hypermetropia: $\frac{1}{ N.P. }-\frac{1}{\mathrm{object}}=\frac{1}{f}=P$

(ix) Limit of resolution for microscope $=\frac{1.22\lambda }{2a\sin \theta }=\frac{1}{\mathrm{resolving} \mathrm{power}}$

(x) Limit of resolution for telescope $=\frac{1.22\lambda }{a}=\frac{1}{\mathrm{resolving} \mathrm{power}}$