EASY

Earn 100

What is the analogue of mass in rotational motion ?

50% studentsanswered this correctly

Important Questions on Rotational Motion

HARD

Physics>Mechanics>Rotational Motion>Moment of Inertia

Moment of inertia of an equilateral triangular lamina

A B C

, about the axis passing through its centre

O

and perpendicular to its plane is

I_{0}

as shown in the figure. A cavity

D E F

is cut out from the lamina, where

D, E, F

are the mid points of the sides. Moment of inertia of the remaining part of lamina about the same axis is:

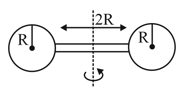
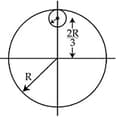
Question Image

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

From a solid sphere of mass

M

and radius

R

, a cube of the maximum possible volume is cut. Moment of inertia of cube about an axis passing through its centre and perpendicular to one of its faces is:

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

A Wheel of moment of inertia

2 k g m^{2}

is rotating about an axis passing through centre and perpendicular to its plane at a speed

60 rad s^{- 1}

. Due to friction, it comes to rest in

5 \min

. The angular momentum of the wheel three minutes before it stops rotating is

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

Two identical spherical balls of mass $M$ and radius $R$ each are stuck on two ends of a rod of length $2 R$ and mass $M$ (see figure). The moment of inertia of the system about the axis passing perpendicularly through the centre of the rod is

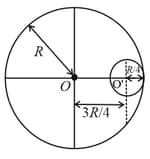
Question Image

EASY

Physics>Mechanics>Rotational Motion>Moment of Inertia

Consider a thin uniform square sheet made of a rigid material. If its side is

a

, mass m and moment of inertia

I

about one of its diagonals, then:

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

A circular hole of radius

\frac{R}{4}

is made in a thin uniform disc having mass and radius

R

, as shown in figure. The moment of inertia of the remaining portion of the disc about an axis passing through the point

O

and perpendicular to the plane of the disc is-
Question Image

EASY

Physics>Mechanics>Rotational Motion>Moment of Inertia

The moment of inertia of a uniform cylinder of length

l

and radius

R

about its perpendicular bisector is

I .

What is the ratio

l / R

such that the moment of inertia is minimum?

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

A solid sphere of mass

M

and radius

R

is divided into two unequal parts. The first part has a mass of

\frac{7 M}{8}

and is converted into uniform disc of radius

2 R

. The second part is converted into a uniform solid sphere. Let

I_{1}

be the moment of inertia of the disc about its axis and

I_{2}

be the moment of inertia of the new sphere about its axis. The ratio

I_{1} / I_{2}

is given by:

EASY

Physics>Mechanics>Rotational Motion>Moment of Inertia

Three identical spherical shells, each of mass $m$ and radius $r$ are placed as shown in the figure. Consider an axis $X X^{'}$ which is touching the two shells and passing through the diameter of the third shell. The moment of inertia of the system consisting of these three spherical shells about $X X^{'}$ axis is:

Question Image

EASY

Physics>Mechanics>Rotational Motion>Moment of Inertia

A thin circular disk is in the

x y

plane as shown in the figure. The ratio of its moment of inertia about

z

and

z'

axes will be:
Question Image

HARD

Physics>Mechanics>Rotational Motion>Moment of Inertia

Seven identical circular planar disks, each of mass M and radius R are welded symmetrically as shown. The moment of inertia of the arrangement about the axis normal to the plane and passing through the point P is:
Question Image

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

The moment of inertia of a solid sphere, about an axis parallel to its diameter and at a distance of

x

from it, is

I (x) .

Which one of the graphs represents the variation of

I (x)

with

x

correctly?

HARD

Physics>Mechanics>Rotational Motion>Moment of Inertia

From a uniform circular disc of radius R and mass 9 M, a small disc of radius

\frac{R}{3}

is removed as shown in the figure. The moment of inertia of the remaining disc about an axis perpendicular to the plane of the disc and passing through centre of disc is:

Question Image

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

Let the moment of inertia of a hollow cylinder of length

30 cm

(inner radius

10 cm

and outer radius

20 cm

), about its axis be

I

. The radius of a thin cylinder of the same mass such that its moment of inertia about its axis is also

I,

is:

HARD

Physics>Mechanics>Rotational Motion>Moment of Inertia

A thin circular plate of mass

M

and radius

R

has its density varying as

ρ (r) {=ρ}_{0} r

with

ρ_{0}

as constant and

r

is the distance from its centre. The moment of Inertia of the circular plate about an axis perpendicular to the plate and passing through its edge is

I = a M R^{2}

. The value of the coefficient

a

is:

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

A thin disc of mass

M

and radius

R

has mass per unit area

σ (r) = k r^{2}

where

r

is the distance from its centre. Its moment inertia about an axis going through its centre of mass and perpendicular to its plane is:

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

A circular disc of radius

b

has a hole of radius

a

at its centre(see figure). If the mass per unit area of the disc varies as

(\frac{σ_{0}}{r})

then, the radius of gyration of the disc about its axis passing through the center is
Question Image

EASY

Physics>Mechanics>Rotational Motion>Moment of Inertia

A light rod of length,

l

has two masses,

m_{1}

and

m_{2}

attached to its two ends. The moment of inertia of the system about an axis perpendicular to the rod and passing through the centre of mass is

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

The moment of inertia of a thin uniform rod of length

L

and mass

M

about an axis passing through a point at a distance of

\frac{L}{3}

from one of its ends and perpendicular to the rod is

MEDIUM

Physics>Mechanics>Rotational Motion>Moment of Inertia

From a disc of radius

R

and mass

M

, a circular hole of diameter

R

, whose rim passes through the centre is cut. What is the moment of inertia of the remaining part of the disc about a perpendicular axis, passing through the centre?

What is the analogue of mass in rotational motion ?

Important Questions on Rotational Motion

Learn and Prepare for any exam you want !