What is the difference between a parallelogram and a kite?

In Figure, $BDEF$ and $DCEF$ are each a parallelogram. Is it true that $\mathrm{BD}=\mathrm{DC}$?

Why or why not?

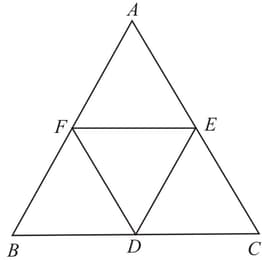
$ABCD$ is a parallelogram in which $\angle A=110°$. Find the measure of each of the angles $\angle B, \angle C$ and $\angle D$.

Given a parallelogram $\mathrm{A}\mathrm{B}\mathrm{C}\mathrm{D}.$ Complete the statement along with the definition or property used.

 $\mathrm{A}\mathrm{D}=$ _____________

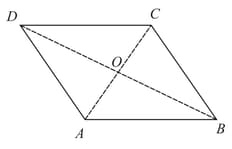
Write ‘T’ for true and ‘F’ for false of each of the following:

i. The diagonals of a parallelogram are equal.

ii. The diagonals of a rectangle are perpendicular to each other.

iii. The diagonals of a rhombus bisect each other at right angles.

iv. Every rhombus is a kite.

Two adjacent angles of a parallelogram are equal. What is the measure of each of these angles?

In Figure, $ABCD$ and $AEFG$are parallelograms. If $\angle C={55}^{\mathrm{o}}$, what is the measure of $\angle F$?

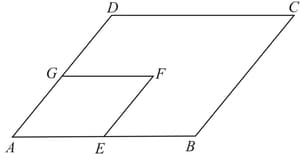
The sum of two opposite angles of a parallelogram is $130°$. Find the measure of each of its angles.

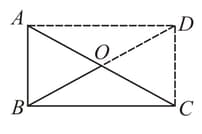
Two adjacent angles of a parallelogram are in the ratio $4:5$. Find the measure of each of its angles.

Two adjacent angles of a parallelogram are $(3x-4)°$ and $(3x+16)°$. Find the value of $x$ and hence find the measure of each of its angles.

Two adjacent angles of a parallelogram are the ratio $2:3$. Find the measure of each of its angles.