HARD

Earn 100

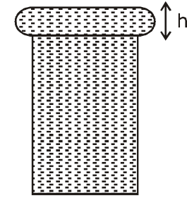
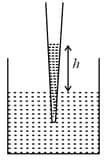
When water is filled carefully in a glass, one can fill it to a height $h$ above the rim of the glass due to the surface tension of water. To calculate $h$ just before water starts flowing, model the shape of the water above the rim as a disc of thickness $h$ having semicircular edges, as shown schematically in the figure. When the pressure of water at the bottom of this disc exceeds what can be withstood due to the surface tension, the water surface breaks near the rim and water starts flowing from there. If the density of water, its surface tension and the acceleration due to gravity are $10^{3} kg m^{- 3}, 0.07 N m^{- 1}$ and $10 m s^{- 2},$ respectively, the value of $h$ is $\sqrt{k} mm$ . Find the value of $k$ .

(Consider the radius of the disc to be very large compared to the radius of the semicircular edges)

50% studentsanswered this correctly

Important Questions on Viscosity and Surface Tension

MEDIUM

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

A big water drop is formed by the combination of

n

small water drops of equal radii. The ratio of the surface energy of

n

drops to the surface energy of the big drop is

EASY

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

Three liquids of densities,

ρ_{1}, ρ_{2}

and

ρ_{3}

(with,

ρ_{1} > ρ_{2} > ρ_{3})

, having the same value of surface tension,

T

, rise to the same height in three identical capillaries. The angles of contact,

θ_{1}, θ_{2}

and

θ_{3}

obey

MEDIUM

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

The following observations were taken for determining surface tension

T

of water by capillary method:

diameter of capillary,

D = 1.25 \times 10^{- 2} m

rise of water,

h = 1.45 \times 10^{- 2} m

Using

g = 9.80 m s^{- 2}

and the simplified relation

T = \frac{r h g}{2} \times 10^{3} N m^{- 1}

the possible error in surface tension is closest to:

MEDIUM

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

A capillary tube is immersed vertically in water and the height of the water column is

x

. When this arrangement is taken into a mine of depth d, the height of the water column is

y

. If R is the radius of earth, the ratio

\frac{x}{y}

is :

HARD

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

Assume that a drop of a liquid evaporates by a decrease in its surface energy so that its temperature remains unchanged. The minimum radius of the drop for this to be possible is. (The surface tension is

T

, the density of the liquid is

ρ

and

L

is its latent heat of vaporisation.)

EASY

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

A soap bubble of radius

r

is blown up to form a bubble of radius

2 r

under isothermal conditions. If

σ

is the surface tension of the soap solution, the energy spent in the process is

EASY

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

A rectangular film of liquid is extended from

(4 cm \times 2 cm)

(5 cm \times 4 cm)

. If the work done is

3 \times 10^{- 4} J

, the value of the surface tension of the liquid is

MEDIUM

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

The ratio of surface tensions of mercury and water is given to be

7.5

, while the ratio of their densities is

13.6

. Their contact angles, with glass, are close to

135 °

and

0 °

, respectively. If it is observed that mercury gets depressed by an amount

h

in a capillary tube of radius

r_{1}

, while water rises by the same amount

h

in a capillary tube of radius

r_{2}

, then the ratio

\frac{r_{1}}{r_{2}}

is close to

HARD

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

If two glass plates have water between them and are separated by very small distance (see figure), it is very difficult to pull them apart. It is because the water in between forms cylindrical surface on the side that gives rise to lower pressure in the water in comparison to atmosphere. If the radius of the cylindrical surface is R and surface tension of water is T then the pressure in water between the plates is lower by:

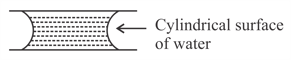
Question Image

MEDIUM

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

Consider a bowl filled with water on which some black paper powder has been sprinkled uniformly. Now a drop of liquid soap is added at the center of the surface of the water. The picture of the surface immediately after this will look like

HARD

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

A large number of liquid drops each of radius

r

coalesce to form a single drop of the radius

R

. The energy released in the process is converted into kinetic energy of the big drop so formed. The speed of the big drop is (given surface tension of the liquid

T

, density

ρ

)

EASY

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

The radius

R

of the soap bubble is doubled under isothermal condition. If

T

be the surface tension of the soap bubble, the work done in doing so is given by

EASY

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

A glass capillary tube is of the shape of a truncated cone with an apex angle

α

so that its two ends have cross-sections of different radii. When dipped in water vertically, the water rises in it to a height

h

, where the radius of its cross-section is

b

. If the surface tension of water is

S

, its density is

ρ

, and its contact angle with glass is

θ

, then the value of

h

will be (

g

is the acceleration due to gravity)

Question Image

EASY

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

When one end of the capillary is dipped in water, the height of the water column is

h

. The upward force of

105 dyne

due to surface tension is balanced by the force due to the weight of the water column. The inner circumference of the capillary is
(Surface tension of water

= 7 \times 10^{- 2} N m^{- 1})

EASY

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

A soap bubble having radius of

1 m m

is blown from a detergent solution having a surface tension of

2.5 \times 10^{- 2} N m^{- 1} .

The pressure inside the bubble equals at a point

Z_{0}

below the free surface of water in a container. Taking

g = 10 m s^{- 2},

density of water

= 10^{3} k g m^{- 3},

the value of

Z_{0}

EASY

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

Which one of the following statement is correct?

EASY

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

' M'

is the mass of water that rises in a capillary tube of radius

' r'

, then mass of water which will rise in a capillary tube of radius

' 2 r'

is:

HARD

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

A small soap bubble of radius 4cm is trapped inside another bubble of radius 6cm without any contact. Let

P_{2}

be the pressure inside the inner bubble and

P_{0}

, the pressure outside the outer bubble. Radius of another bubble with pressure difference

P_{2} - P_{0}

between its inside and outside would be:

EASY

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

Water rises to a height 'h' in capillary tube. If the length of capillary tube above the surface of water is made less than 'h', then:

MEDIUM

Physics>Mechanics>Viscosity and Surface Tension>Surface Tension

A soap bubble, blown by a mechanical pump at the mouth of a tube increases in volume with time at a constant rate. The graph that correctly depicts the time dependence of pressure inside the bubble is given by:

Important Questions on Viscosity and Surface Tension

Learn and Prepare for any exam you want !