EASY

Earn 100

Why do induced currents oppose the change in magnetic flux that causes them?

Important Questions on Electromagnetic Induction

HARD

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A long solenoid of radius

R

carries a time

(t)

dependent current

I (t) = I_{0} t (1 - t)

. A ring of radius

2 R

is placed coaxially near its middle. During the time interval

0 \leq t \leq 1,

the induced current

(I_{R})

and the induced

E M F (V_{R})

in the ring change as:

HARD

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A circular insulated copper wire loop is twisted to form two loops of area

A

and

2 A

as shown in the figure. At the point of crossing the wires remain electrically insulated from each other. The entire loop lies in the plane (of the paper). A uniform magnetic field

\vec{B}

points into the plane of the paper. At

t = 0

, the loop starts rotating about the common diameter as axis with a constant angular velocity in the magnetic field. Which of the following options is/are correct?
Question Image

MEDIUM

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A very long solenoid of radius

R

is carrying current

I (t) = k t e^{- α t} (k > 0),

as a function of time

(t \geq 0) .

Counterclockwise current is taken to be positive. A circular conducting coil of radius

2 R

is placed in the equitorial plane of the solenoid and concentric with the solenoid. The current induced in the outer coil is correctly depicted, as a function of time, by:

MEDIUM

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

The relation between the charge flow

ΔQ

through the circuit of resistance

r

and the change in the magnetic flux

{Δϕ}_{B}

EASY

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

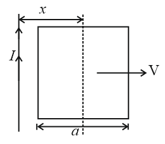
In a coil of resistance $100 Ω$ , a current is induced by changing the magnetic flux through it as shown in the figure. The magnitude of change in flux through the coil is:
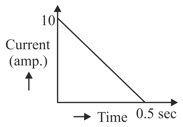
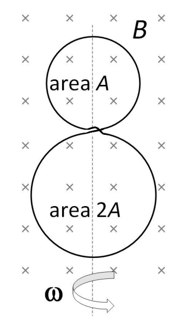
Question Image

MEDIUM

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A planar loop of wire rotates in a uniform magnetic field. Initially, at

t = 0

, the plane of the loop is perpendicular to the magnetic field. If it rotates with a period of

10 s

about an axis in its plane then the magnitude of induced emf will be maximum and minimum, respectively at:

MEDIUM

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

800

turn coil of the effective area

0.05 m^{2}

is kept perpendicular to the magnetic field

5 \times 10^{- 5} T .

When the plane of the coil is rotated by

90^{o}

around any of its coplanar axis in

0.1 s,

the emf induced in the coil will be:

EASY

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

The magnetic flux linked with a coil (in $Wb$ ) is given by the equation $ϕ = 5 t^{2} + 3 t + 16$ . The magnitude of induced emf in the coil at the fourth second will be:

MEDIUM

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

The figure shows a bar magnet and a metallic coil. Consider four situations. (I) Moving the magnet away from the coil. (II) Moving the coil towards the magnet. (III) Rotating the coil about the vertical diameter. (IV) Rotating the coil about its axis.

Question Image

An emf in the coil will be generated for the following situations.

EASY

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A long solenoid of diameter

0.1 m

has

2 \times 10^{4}

turns per meter. At the centre of the solenoid, a coil of

100

turns and radius

0.01 m

is placed with its axis coinciding with the solenoid axis. The current in the solenoid reduces at a constant rate to

0 A

from

4 A

0.05 s

. If the resistance of the coil is

10 π^{2} Ω,

the total charge flowing through the coil during this time is

MEDIUM

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A circular coil of radius

10 cm

is placed in a uniform magnetic field of

3.0 \times 10^{- 5} T

with its plane perpendicular to the field initially. It is rotated at constant angular speed about an axis along the diameter of coil and perpendicular to magnetic field so that it undergoes half of rotation in

0.2 s

. The maximum value of EMF induced (in

μV

) in the coil will be close to the integer....

MEDIUM

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A uniform magnetic field is restricted within a region of radius,

r .

The magnetic field changes with time at a rate,

\frac{d \vec{B}}{d t}

. Loop one of radius

R > r

encloses the region,

r

and loop two of radius,

R

is outside the region of magnetic field as shown in the figure below. Then the emf generated is

Question Image

EASY

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A small bar magnet is moved through a coil at constant speed from one end to the other. Which of the following series of observations will be seen on the galvanometer $G$ attached across the coil?

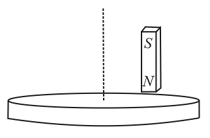
Question Image

Three positions shown describe: (a) the magnet's entry (b) magnet is completely inside and (c) magnet's exit.

MEDIUM

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

At time

t = 0

magnetic field of

1000 G a u s s

is passing perpendicularly through the area defined by the closed loop shown in the figure. If the magnetic field reduces linearly to

500 G a u s s,

in the next

5 s,

then induced

E M F

in the loop is:
Question Image

HARD

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A light disc made of aluminium (a nonmagnetic material) is kept horizontally and is free to rotate about its axis as shown in the figure. A strong magnet is held vertically at a point above the disc away from its axis. On revolving the magnet about the axis of the disc, the disc will (figure is schematic and not drawn to scale)

Question Image

EASY

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

The magnetic flux through a coil varies with time as

ϕ = 5 t^{2} + 6 t + 9

. The ratio of emf at

t = 3 s

t = 0 s

will be

EASY

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A conducting circular loop is placed in a uniform magnetic field,

B = 0.025 T

with its plane perpendicular to the loop. The radius of the loop is made to shrink at a constant rate of

1 mm s^{- 1}

. The induced emf when the radius is

2 cm

, is

HARD

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A conducting metal circular-wire-loop of radius

r

is placed perpendicular to a magnetic field which varies with time as

B = B_{0} e^{- \frac{t}{τ}},

where

B_{0} and τ

are constants at time

t = 0

. If the resistance of the loop is

R

, then the heat generated in the loop after a long time

(t \to \infty)

EASY

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A coil of cross-sectional area

A

having

n

turns is placed in a uniform magnetic field

B

. When it is rotated with an angular velocity

ω,

the maximum e.m.f. induced in the coil will be:

HARD

Physics>Electricity and Magnetism>Electromagnetic Induction>Faraday's Laws of Induction

A conducting square frame of side

a

and a long straight wire carrying current

I

are located in the same plane as shown in the figure. The frame moves to the right with a constant velocity

V

. The e.m.f induced in the frame (when the centre of the frame is at a distance

x

from the wire) will be proportional to :
Question Image

Why do induced currents oppose the change in magnetic flux that causes them?

Important Questions on Electromagnetic Induction

Learn and Prepare for any exam you want !