Angle Subtended by a Chord at a Point
Angle Subtended by a Chord at a Point: Overview
The topic states that if the angles subtended by the two chords of congruent circles at the corresponding centers are equal then the chords are equal.
Important Questions on Angle Subtended by a Chord at a Point
In the given figure is the centre of the circle and are equal chords. If . If , then find the value of .
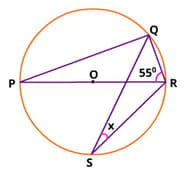
The given figure shows a circle with centre . Also, and . If , then find the value of .
is an equilateral triangle and are two points on the circle. Find and .

In the given figure is the centre of the circle and are equal chords. If . Find the angles of the .
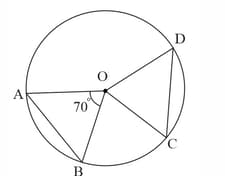
In the figure, . If the measure of . Find .

In the figure given below, and , find and ,
In the figure given below, if and ,find angle (in degree).
is a tangent to the circle at If and calculate .
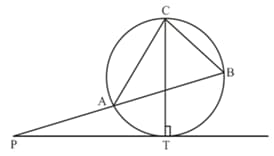
is a tangent to the circle at If and calculate .
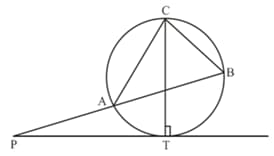
In the given figure, and are inscribed in a circle such that and Then equal to
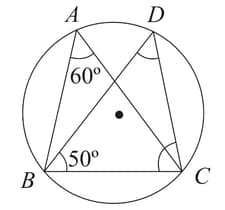
Equal chords of a circle subtend equal angles at
In the following figure, is the tangent to the circle at is the diameter and is the centre of the circle. If and calculate
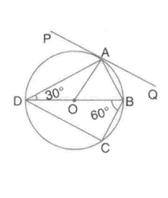
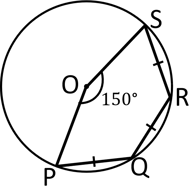
In the above figure, O is the centre of the circle. The value of x is _____ degrees.
Bisectors of vertex angles and of a triangle intersect its circumcircle at the points and respectively. Prove that angle
In the given figure, is a tangent to the circle at and are bisectors of and If prove that is diameter of the circle.

is in the following figure, where and are points on a circle with center . find

Draw a circle of radius cm. Draw two chords of length cm. Measure the angles made by each chord at the centre. Are the values of both the angles equal?
is the center of the circle in the figure. The radius is perpendicular to the diameter . If is a point on the circle, then find and .
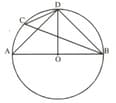
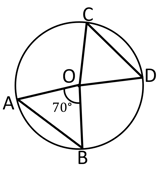
is the center of the circle in the figure. Chord Chord and then the value of is:
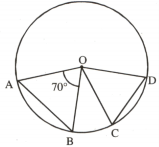
The length of a chord of a circle is equal to its radius. Give the value of the angle made by the chord at the centre.