Friction

Consider a car moving along a straight horizontal road with speed of $72 \mathrm{km} {\mathrm{hr}}^{-1}$  . If the coefficient of static friction between the tyres and the road is $0.5$, the shortest distance in which the car can be stopped is  (taking $g=10 \mathrm{m} {\mathrm{s}}^{-2}$)

A conveyor belt is moving at a constant speed of  $2 \text{m }{\text{s}}^{-1}$.  A box is gently dropped on it. The coefficient of friction between them is  $\mu =\mathrm{0.5.}$  The distance that the box will move relative to belt before coming to rest on it taking  $g=10\mathrm{m}{\mathrm{s}}^{-2}$, is

A block of mass $0.1 \mathrm{kg}$ is held against a wall by applying a horizontal force of $5 \mathrm{N}$ on the block. If the coefficient of friction between the block and the wall is $0.5,$ the magnitude of the frictional force acting on the block is (take $g=9.8 \mathrm{m} {\mathrm{s}}^{-2}$)

A block of mass $2 \mathrm{kg}$ rests on a rough inclined plane making an angle of $30º$ with the horizontal. The coefficient of static friction between the block and the plane is $0.7.$ The frictional force on the block is

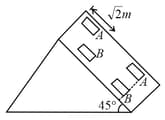
Two block $A$ and $B$ of equal masses are placed on a rough inclined plane as shown in figure. When (and where) will the two blocks come on the same line on the inclined plane if they are released simultaneously? Initially the block $A$ is $\sqrt{2}m$ behind the block $B$. Co-efficient of kinetic friction for the blocks $A$ and $B$ are $0.2$ and $0.3$ respectively $\left(g=10m{s}^{-2}\right)$

In the figure masses $m_1, m_2$ and $M$ are $20 \mathrm{kg}, 5 \mathrm{kg}$ and $50 \mathrm{kg}$ respectively. The coefficient of friction between $M$ and ground is zero. The coefficient of friction between $m_1$ and $M$ and that between $m_2$ and ground is $0.3.$ The pulleys and the strings are massless. The string is perfectly horizontal between $P_1$ and $m_1$ and also between $P_2$ and $m_2.$ The string is perfectly vertical between $P_1$ and $P_2.$ An external horizontal force $F$ is applied to the mass $M.$ Take $g=10 {\mathrm{ms}}^{-2}.$

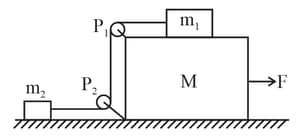
(a) Draw a free-body diagram for mass $M,$ clearly showing all the forces.
(b) Let the magnitude of the force of friction between $m_1$ and $M$ be $f_1$ and that between $m_2$ and ground be $f_2.$ For a particular $F,$ it is found that $f_1=2f_2.$ Find $f_1$ and $f_2.$ Write equations of motion of all the masses. Find $F,$ tension in the string and acceleration of the masses.

Block $A$ of mass $m$ and block $B$ of mass $2m$ are placed on a fixed triangular wedge by means of a massless inextensible string and a frictionless pulley as shown in figure. The wedge is inclined at $45°$ to the horizontal on both sides. The coefficient of friction between block $A$ and the wedge is $\frac{2}{3}$ and that between block $B$ and the wedge is $\frac{1}{3}$. If the system of $A$ and $B$ is released from rest, find the acceleration of $A \& B$.

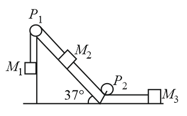
Masses  $M_1,M_2\mathrm{and}{M}_3$ are connected by strings of negligible mass which pass over massless and frictionless pulleys  $P_1\mathrm{and}{P}_2$  as shown in the figure. The masses move such that the portion of the string between  $P_1\mathrm{and}{P}_2$ is parallel to the inclined plane and portion of the string between  $P_2\mathrm{and}{M}_3$ is horizontal. The masses  $M_2\mathrm{and}{M}_3$  are $4 \mathrm{kg}$ each and coefficient of kinetic friction between the masses and the surfaces is 0.25. The inclined plane makes an angle of  $37°$  with the horizontal. If the mass  $M_1$  moves downwards with a uniform velocity, find the tension in the horizontal portion of the string.  $\left(g=9.8 m s^{-2}, \sin 37°=\frac{3}{5}\right)$

Two blocks connected by a massless string slide down an inclined plane having an angle of inclination of  $37°.$  The masses of the two blocks are  $M_1=4kg$  and  $M_2=2kg$  respectively and the coefficients of friction of  $M_1$ and  $M_2$ with the inclined plane are 0.75 and 0.25 respectively. Assuming the string to the taut, find the tension in the string.  $\left(\sin 37°=0.6,\cos 37°=0.8\right)$

Two blocks connected by a massless string slide down an inclined plane having an angle of inclination of $37°.$ The masses of the two blocks are $M_1=4 \mathrm{kg}$ and $M_2=2 \mathrm{kg}$ respectively and the coefficients of friction of $M_1$ and $M_2$ with the inclined plane are $0.75$ and $0.25$ respectively. Assuming the string to be taut, find the common acceleration of two masses. $\left(\sin 37°=0.6, \cos 37°=0.8\right)$

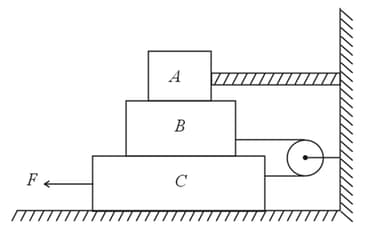
In the diagram shown, the blocks$\mathrm{A}, \mathrm{B} \mathrm{and} \mathrm{C}$ weight, $3 \mathrm{kg}, 4 \mathrm{kg} \mathrm{and} 5 \mathrm{kg}$ respectively. The coefficient of sliding friction between any two surface is $0.25$. A is held at rest by a massless rigid rod fixed to the wall while $\mathrm{B}$ and $\mathrm{C}$ are connected by a light flexible cord passing around a frictionless pulley. Find the force $F$ necessary to drag $\mathrm{C}$ along the horizontal surface to the left at constant speed. Assume that the arrangement shown in the diagram, $\mathrm{B} \mathrm{on} \mathrm{C} \mathrm{and} \mathrm{A} \mathrm{on} \mathrm{B}$, is maintained all through.  $(g=9.8\mathrm{m}{\mathrm{s}}^{-2})$

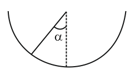
An insect crawls up a hemispherical surface very slowly (see the figure). The coefficient of friction between the insect and the surface is $\frac{1}{3}$. If the line joining the center of the hemispherical surface to the insect makes an angle $\alpha$ with the vertical, the maximum possible value of $\alpha$ is given by,

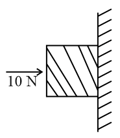
A horizontal force of $10 \mathrm{N}$ is necessary to just hold a block stationary against a wall. The coefficient of friction between the block and the wall is $0.2$. The weight of the block is –

The upper half of an inclined plane with inclination $\mathrm{\varphi }$ is perfectly smooth while the lower half is rough. A body starting from rest at the top will again come to rest at the bottom if the coefficient of friction for the lower half is