Toppling

A uniform rectangular box of dimensions $\left(40 \mathrm{cm}\times 20 \mathrm{cm}\right)$ is kept on horizontal smooth surface at a distance $10 \mathrm{m}$ from a wall. A constant force $F$ is applied at the highest point as shown. The minimum time (in $\mathrm{s}$) to reach the wall without causing the box to rotate is:    $\left(g=9.8 \mathrm{m} {\mathrm{s}}^{-2}\right)$

​​​​​​​

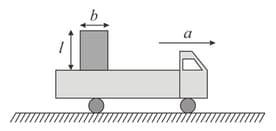
A truck is moving with acceleration $a$. A box of dimensions $l$ and $b$ is kept on it. If the box does not slide, the maximum acceleration for it to remain in equilibrium (with respect to the truck) is

An ice cube of mass $M$ and with sides of length a is sliding without friction with speed $v_0$ when it hits a ridge $O$ at the edge of counter (see figure). This collision causes the cube to tilt as shown. The minimum value of ${\mathrm{v}}_0$ needed for the cube to fall off the table is given by $\sqrt{\mathrm{kag}}.$ Find value of $k.$ (Approximate the answer to nearest integer.)

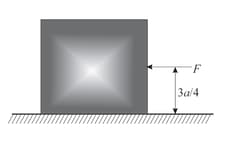
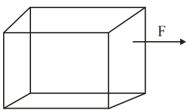
Consider a uniform cubical box of side $a$ on a rough floor that is to be moved by applying minimum possible force $F$ at a point $b$ above its centre of mass (see figure). If the coefficient of friction is $\mu =0.4,$ the maximum possible value of $100\times \frac{\mathrm{b}}{\mathrm{a}}$ for box not to topple before moving is ________

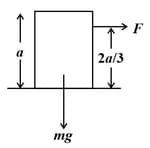
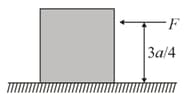
A uniform cube of side a & mass m rests on a rough horizontal table. A horizontal force $F$ is applied normal to one of the faces at a point that is directly above the centre of the face, at a height $3 a/4$ above the base. The minimum value of $F$ for which the cube begins to tip about an edge is -----.(assume that cube does not slide).

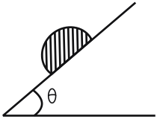
An uniform semi-solid sphere is placed with flat surface on rough inclined plane as shown in the figure. If the friction is large for no sliding, then the minimum angle $\mathrm{\theta }$ at which toppling occur is,

An engineer is designing a conveyor system for loading lay bales into a wagon. Each bale is 0.25 m wide, 0.50 m high, and 0.80 m long (the dimension perpendicular to the plane of the figure), with mass 30.0 kg. The center of gravity of each bale is at its geometrical center. The coefficient of static friction between a bale and the conveyor belt is 0.60, and the belt moves with constant speed. The angle $\text{β}$ of the conveyor is slowly increased. At some critical angle a bale will tip (if it doesn't slip first), and at some different critical angle it will slip (if it doesn't tip first).

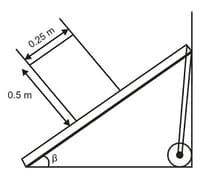
Find the first critical angle (In the same conditions) at which it tips..

A uniform cube of mass $m$ and side $a$ is resting in equilibrium on a rough $45°$ inclined surface. The distance of the point of application of normal reaction measured from the lower edge of the cube is

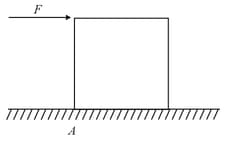
A force $F$ is applied on the top of a cube as shown in the figure. The coefficient of friction between the cube and ground is $\mu$. If  $F$ is gradually increased, then the cube will topple before sliding. Then the range of $\mu$ is

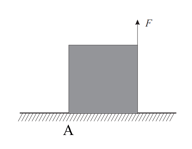
A uniform cube of mass $m$ and side $a$ is placed on frictionless horizontal surface. A vertical force $F=\frac{mg}{4}$ is applied to the edge as shown in figure. Normal reaction effectively acts at a distance   

An equilateral prism of mass $m$ rests on a rough horizontal surface with coefficient of friction $\mu$. A horizontal force $F$ is applied on the prism as shown in the figure. If the coefficient of friction is sufficiently high so that the prism does not slide before toppling, then the minimum force required to topple the prism is

A uniform cube of side $a$ and mass $m$ rests on a rough horizontal table. A horizontal force $F$ is applied normal to one of the faces at a point that is directly above the centre of the face, at a height $\frac{3a}{4}$ above the base. The minimum value of $F$ for which the cube begins to topple about an edge is (assume that cube does not slide).

When the driver of a car suddenly notices a broad wall in front of him, he must:

A uniform rod of mass $m$, and length $l$ is placed on a frictionless horizontal surface. A sudden horizontal impulse $p$, is given to the rod perpendicular to length at a distance $\frac{l}{4}$ from the centre. What will be the angular velocity of the rod ?

On a rough horizontal table, a uniform cube of side$a$ and mass $m$ is resting . If a horizontal force $F$ is applied normal to one of the faces at a point directly above the centre of the face, at a height $\frac{\mathit{3}\mathit{a}}{\mathit{4}}$ above the base, then calculate the minimum value of $F$ for which the cube begins to tip about an edge ?

A uniform cubical box of side $a$ is placed on a rough floor that is to be moved by applying minimum possible force $F$ at a point which is at a distance $b$ above its centre of mass (see figure). If the coefficient of friction is $\mu =0.4,$ the maximum possible value of $100\times \frac{\mathit{b}}{\mathit{a}}$ for which the box will not topple before moving is

Find the maximum height at which $F$ acts, so that sliding and tipping are equally likely to occur.

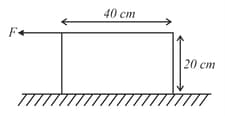
Find the greatest height at which force $F$ may be applied to slide the block without tipping over.

If the friction is sufficient to prevent the block from sliding, then the minimum value of $\mathrm{F}$ for which the cube begins to topple about an edge is

A uniform cubical box of side $a$ is placed on a rough floor and it is to be moved by applying minimum possible force $F$ at a point which is at a distance $b$ above its centre of mass. If the coefficient of friction is $\mu =0.4,$ then what is the maximum possible value of $100\times \frac{b}{a}$ for which the box will not topple before moving?